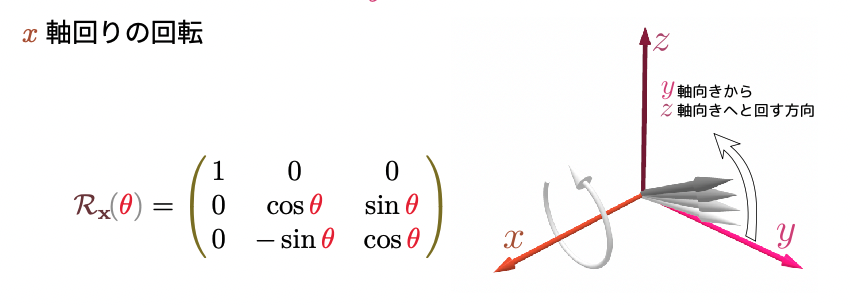すいません、忙してくてレポートの採点が遅れてます。この授業のためのアプリを作るのに時間がかかっているという点もあるので、もう少し待ってください。
前回の感想・コメントシートから
前回の授業の「感想・コメント」の欄に書かれたことと、それに対する返答は、
第8回授業への受講者の感想・コメント
にありますので見ておいてください。
前回までで行列式・逆行列を使って連立方程式を解く話をしました。少し物理よりに話をシフトして、今日は「回転」を行列で表す話をしましょう。
2次元平面でのベクトルの回転
図形で考えるベクトルの回転
以下の話の説明ビデオは↓
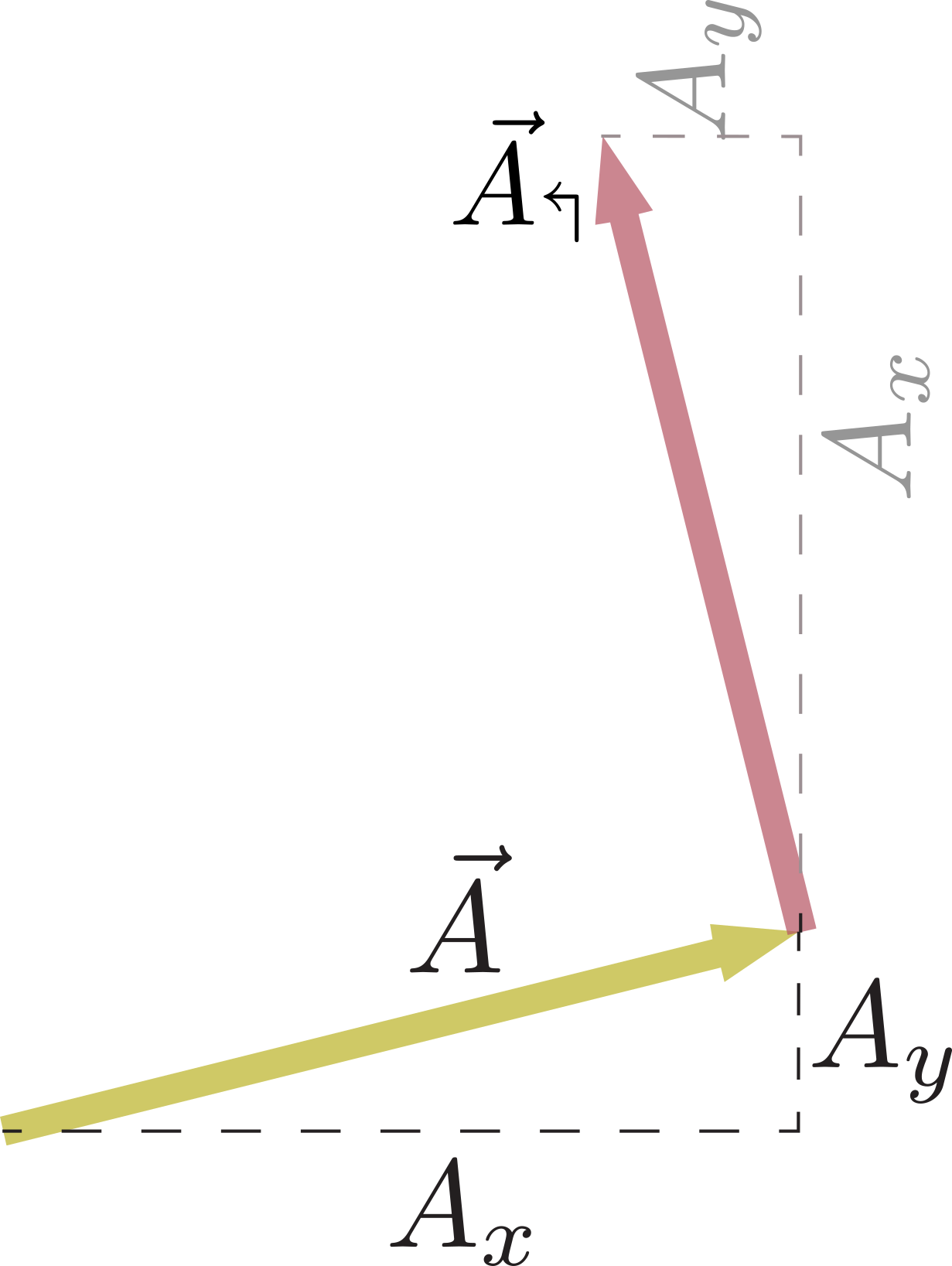
ここでは、2次元平面の上でベクトルを角度だけ回転させたベクトルをどのように作れば(あるいは数式上で表現すれば)よいかということを考えよう。図で描くならば
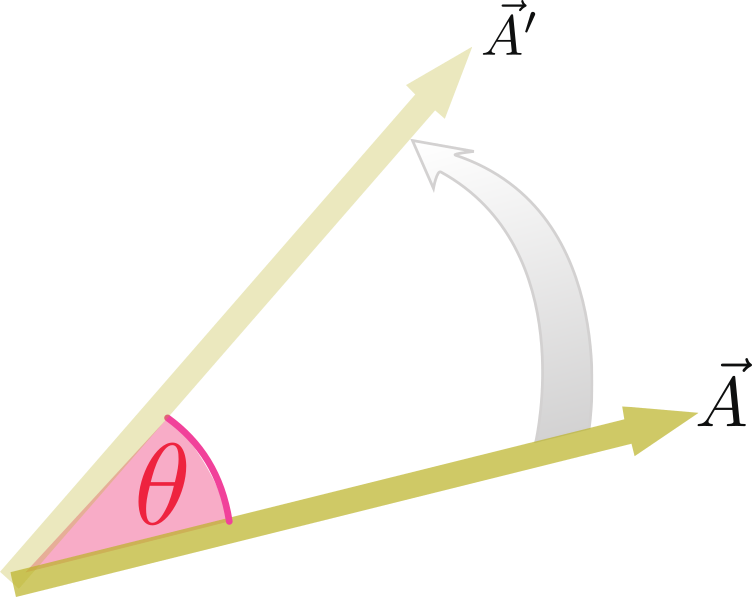
のような状況である。
最初に一つ確認しておくが、この回転の操作をのように表したとき、このは線形写像である。
すなわち、
が成り立つ。ゆえに行列で表現できる。
ここで行う回転は、実際に平面上にあるベクトルが向きを変えるという、物理的現象である。
回転を数式の上ではどのように扱うべきかを解説する。
ベクトルの回転を表現してみよう。 回転を実現するには、
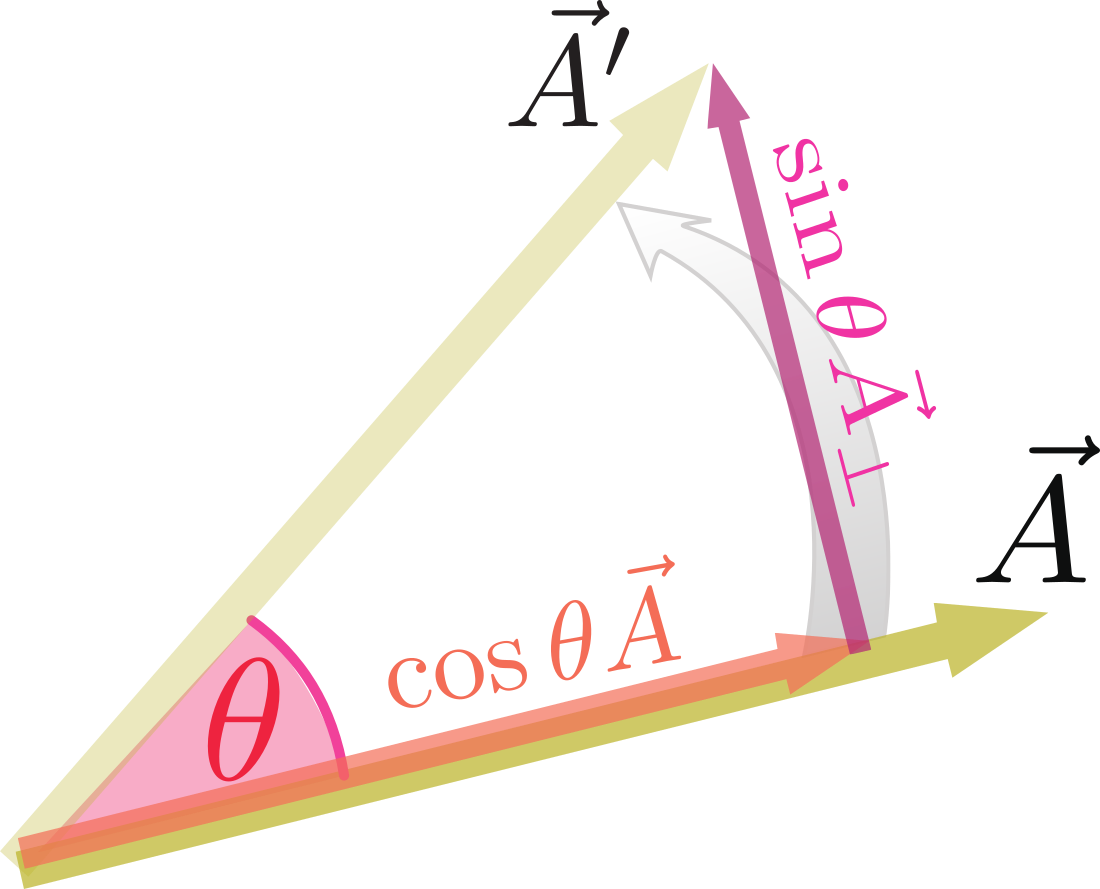
のように、元のベクトルの長さを倍にしたもの()に、元のベクトルを90度(rad)倒して(このベクトルをと書くことにする)長さを倍にした、を足す、という操作を行う。
ここでは90度倒すことを表す記号を「」にした(一般的な記号ではない)。倒す方向は、反時計回り。平面の回転では「正の回転」を反時計回り、「負の回転」を時計回りとすることが多い。北極側から見た地球の自転は反時計回りで、正の回転である。
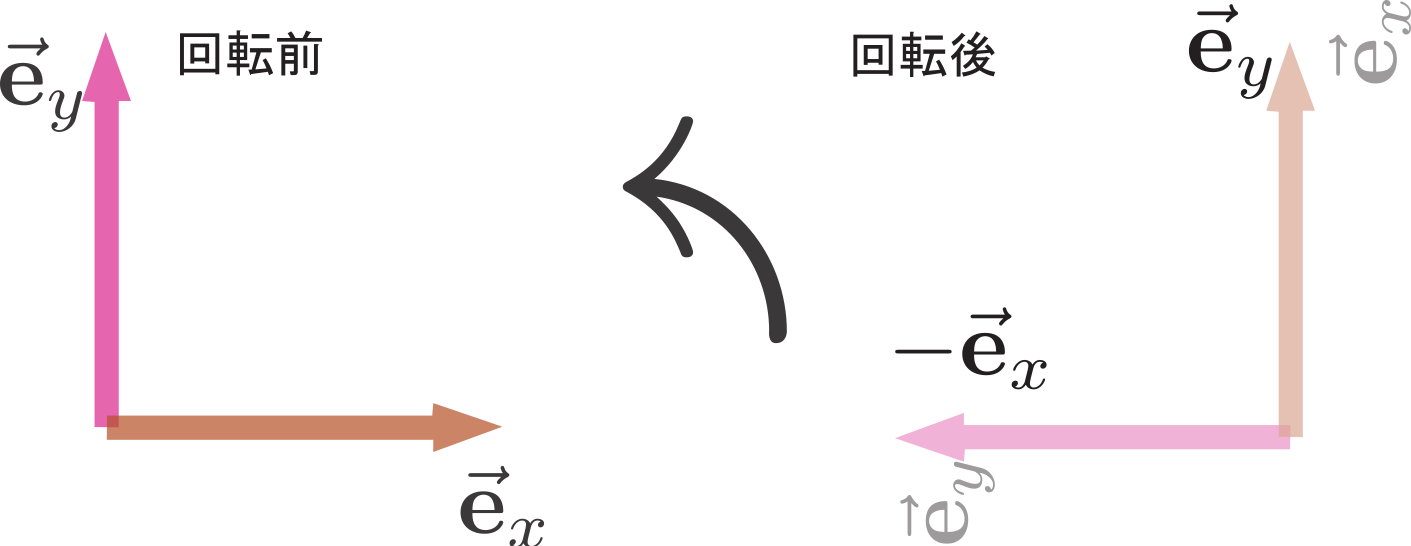
そこでまず反時計周りの90度(rad)回転を考えることにしよう。
の成分を
のように考えることで、「回転後の成分は元の成分()の符号を変えたもの、回転後の成分は元の成分()」という関係がわかる。「回転後のベクトルを、古い座標系でみたときの成分」が並ぶ位置が二つの変換では違うのである。
すなわち成分の変化はであり、回転の結果は
である。
あるいはこれを
のように、という置き換えをした結果ベクトルが回転したと考えてもよい。
とで符号の付く位置が違うことに注意(だが、これでいい)。
こう考えても結果は
となる。
行列で表現すると成分の変化はであり、基底ベクトルの変化はとなることに注意。二つの表現は互いに行列の転置になっている。
の回転は上の通りであったので、角度の回転を「元のベクトルの倍に、の倍を足す」という操作で実現してみると、
となる。
加法定理
角度の回転を行ってから角度の回転を行うという操作は(2次元で考える限り)「角度の回転」と同じ操作である。「行列は操作を表現したもの」なので、「二つの操作の合成」は行列の掛算で計算できる。やってみると、
となって、三角関数の加法定理が出てくる。
また、この結果を見ると、
- 「角度の回転を行ってから角度の回転を行う」
- 「角度の回転を行ってから角度の回転を行う」
が同じものだわかる。これは二つの行列とが可換であることを意味している。このため、2次元の回転は行列で表現されてはいるが、3次元以上に比べれば、かなり単純だとも言える。
成分の変換と基底の変換
成分の変換と基底の変換
以下の話の説明ビデオは↓
数式で回転を表現するには(実は回転に限らずもっと広い範囲の座標変換で言える)、次の図で表す二つの考え方がある。「成分の変換」と「基底の変換」である。
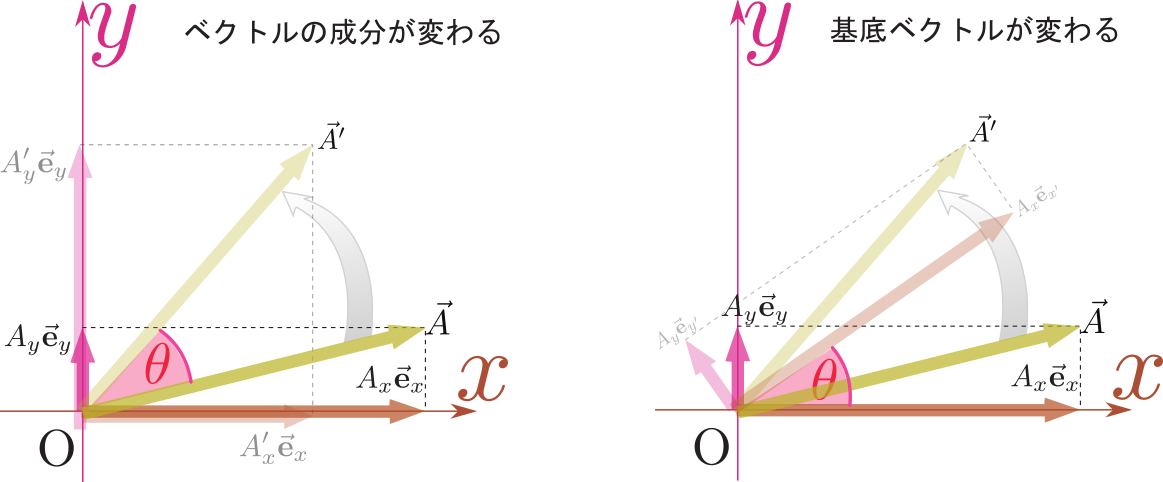
どちらの場合でも、最初のベクトルはと表現されている。が変化することによって回転が実現する、というのが左の図(成分の変換)、の向きが変わることによって回転が実現する、というのが右の図(基底の変換)の考え方である。
前からを掛けることにより、ベクトルが
のようにに変換されるわけだが、この式を
と考えれば成分が変換されたということになるし、
と考えれば基底ベクトルが変換されたということになる。この式は転置して書くと
となるので、成分と基底を列ベクトルで表現することにした場合の、「成分を変換するための行列」と「基底ベクトルを変換するための行列」は互いに転置を取った関係であることに注意しよう。
この関係を不思議に思う人もいるかもしれないが、この関係を不思議に思う人もいるかもしれないが、この二つの変換によってすなわちyがどのように変換されるかを考えると、
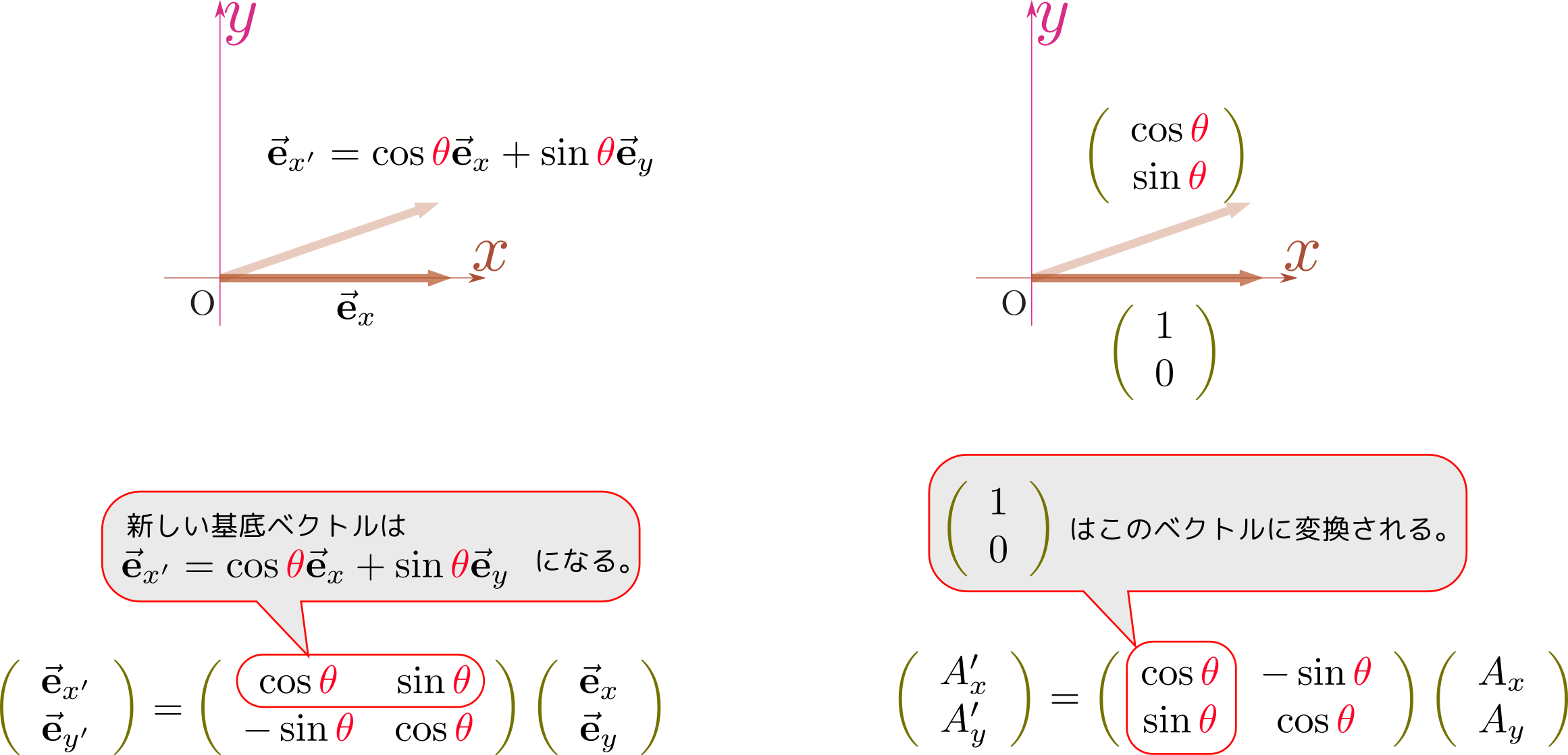
となって、行列が転置された並び方になっていればこそ、この二つの変換が同じ回転を表現できていることがわかる。「回転後のベクトルを、古い座標系でみたときの成分」が並ぶ位置が二つの変換では違うのである。
行列の転置と回転の行列
以下の話の説明ビデオは↓
ここで「行列の転置」について説明しておく。「転置する(縦のものを横にする)」という操作は、行列にとっては
のように、行と列を入れ替える操作である。これにより、行列は行列に変わる。正方行列である場合は転置しても行数と列数は変わらない。
転置という操作はのようにtという上付き添字をつけて表す。転置しても元と同じ行列(を満たす行列)を「対称行列(symmetric matrix)」、逆に転置すると符号が変わる行列(を満たす行列)を「反対称行列(antisymmetric matrix)」と言う。対称行列にしろ反対称行列にしろ、正方行列でなければ意味がない言葉である。
転置が以下の性質を満たすことはすぐにわかる。
最後の4.だけが少し考えないとわからないかもしれないが、行列の掛け算が
であり、これを転置するということは、
とすることだ(このように行列の順番を変えないととの内積を取る形にならない)と気づけば、転置するときに行列の掛け算の順番が入れ替わることは納得できるだろう。
前項で作った角度の回転の行列は、転置するととなり、これは角度の回転の行列である。つまり、はの逆行列である。これは偶然でもなんでもない。行列は、を回した結果の列ベクトルを並べたものと解釈できるからなのである。
この二つのベクトルは「を回した結果」と「を回した結果」であり、このベクトルを並べるとができる。ここで、
のようにしてみると、がすぐわかる(回転の前のがを満たしていたのだから、回転後も満たしているのは当然だ)。
以上は平面でなく任意の次元の「回転を表す行列」に関して言える。一般に、を満たす行列を「直交行列(orthogonal matrix)」と呼ぶ。名前に「直交」が入っているのは「行列を列ベクトルの組とみたとき、列ベクトルは相互に直交している(内積が0)」を意味している。
2次元の直交行列には、では表せないもの(どんなを持ってきても表現できないもの)がある。例を挙げよ。
答えはここにある。考えて答えがわかったらクリック。
たとえば、。これは直交する2本の列ベクトルを並べているが、回転では得られない(上下反転の行列)。
微小角度の回転と生成子
以下の内容の説明ビデオが↓
微小角度の回転の無限回繰り返し
ここでとのテイラー展開
をこの式に代入すると、
のようになるが、この展開の規則性をよくみると、
とまとめることができる。
この式をと書きなおしてからオイラーの式と見比べると、実はという行列が「虚数単位」と同じ役割を果たしていることがわかる。実際、であり、自乗するとという性質を持っているのである。
確かに、
という式を見ると、複素平面上ではを掛けるという操作が角度の回転であることがわかる。
指数関数のテイラー展開
を思い出し、が行列であってもこの式が使えると考えると、
と書くことができる。
指数関数は、
のように、極限を使って定義することもできた。同様に、
と考えることもできる。ここに現れた行列の意味は、元のベクトル元のベクトルを回して長さを倍したベクトル}という演算であり、これは「微小角度の回転」に対応している。つまり「微小角度の回転」を回繰り返すことで角度の回転を作っている。
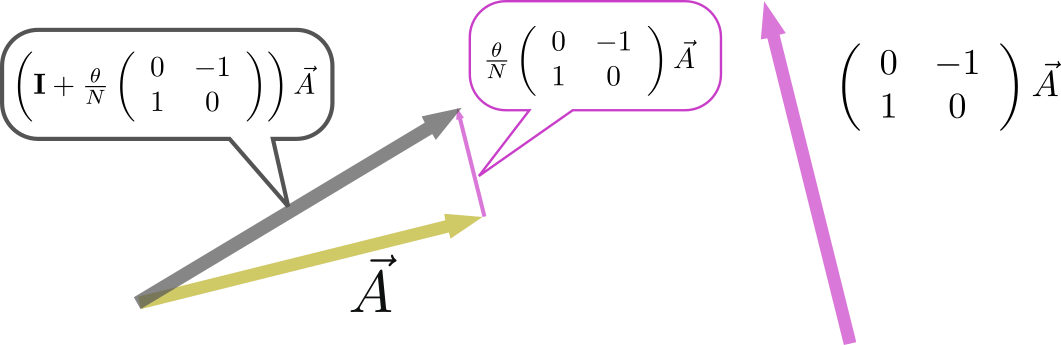
後での都合上の肩にを入れて、
のように回転行列を表したときの行列
を、「回転の生成子」と呼ぶ。
これに限らず「微小変換」を考えて、その微小変換が(微小なパラメータをとして)
と書けるとき、を変換の「生成子(generator)」と呼ぶ。パラメータが微小でない場合は、変換はとなる。
3次元回転
3次元回転の行列による表現
以下の話の説明ビデオは↓
3次元の回転を考えるために、以下に示すような3種類の2次元回転をまず考えることにしよう。以下では
と書いたとき(基底ベクトルを列ベクトルとしたとき)の行列について考えていくことにする。わざわざこう書いたのは、
と書いたとき(成分ベクトルを列ベクトルとしたとき)の行列と区別するためである(実は)。
本講義では、成分を変換する行列は、基底を変換する行列はとする。
以下で説明する、三つの3次元回転をアプリで説明したビデオ(アプリは下の方にリンクがあります)
まず、軸周りの回転は平面での回転だから、2次元の回転と同じになる。

のように行列で表現される。成分に対しては何もしないので、その部分は1になっている。念のため行列を使わずに書き下すと
である。
同様に、軸回りの回転と軸回りの回転を表現すると以下のようになる。
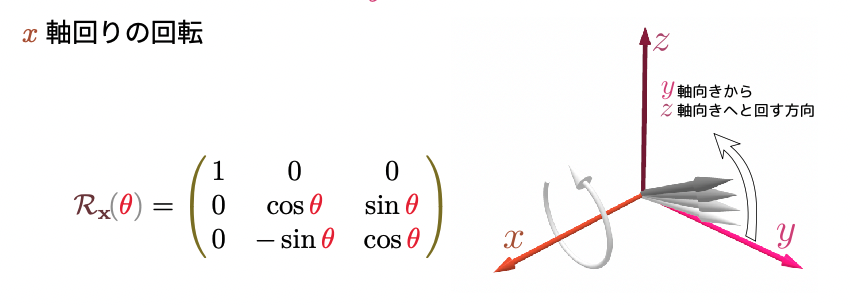
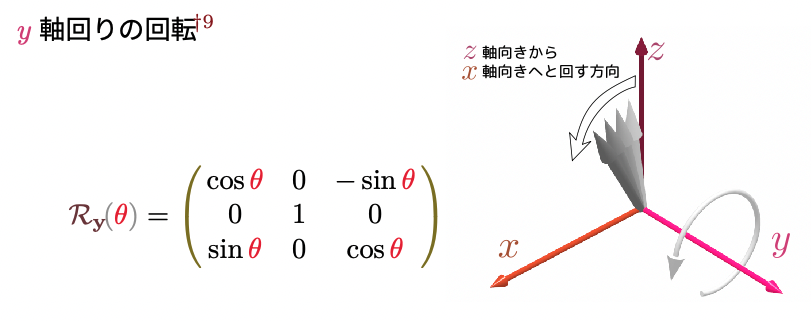
この軸回りの回転だけ符号が逆なのでは?---と不思議に思う人がいるのだが、軸回りの回転は「の回転」だということを考えると、これでいいことがわかる。
以下で説明している「回転は交換しない」ことをアプリで確認しているビデオは↓
使っているアプリは、
ここで重要なことは、これらの行列は互いに可換ではないということである。具体的に計算してみると、
と
である。実際に何かを回してみても、
- 軸回りに回してから軸回りに回す
- 軸回りに回してから軸回りに回す
の結果は全く違う。
オイラー角
さてここで当然「斜めの軸で回すのはどう表現するのか」という疑問が湧くことと思う。この疑問に関しては「上の回転を組み合わせれば、すべての回転は表現できるのでは?」という解答がありえるだろう。
オイラー角については、アプリで説明しよう。以下のアプリをやってみよう。説明は簡単なビデオがつけてあるが、下の文章も合わせて読んで、どういう変換をしているかを確認しよう。
「組み合わせる」ということはもちろん、行列の掛算を行うということである。よく使われるのが「Euler角(Euler Angle)」(Eulerはカタカナ表記では「オイラー」)という3つのパラメータを使った行列の掛算で、基底の変換なら
のような3つの行列の積で回転を表現する。この順の行列の積を使う場合は「ZXZのオイラー角による変換」と呼ぶ。行列の組み合わせは他にもXYZにしたりすることもある。
この積は具体的に計算すると
となる。基底の変換ではなく成分の変換なら全体を転置すればよいので、
となる。この行列は
と表現することもできる(ゆえに成分の変換として考えるときは、の順番が逆になる(成分の変換と基底の変換、どちらを使っているのかに注意)。
このあたり、細かい計算までフォローしなくてよいが、「3つの回転の組合せで様々な(実はすべての)回転が表現できる」ということをアプリの方で実感しておいてください。
CG(コンピュータグラフィクス)などで物体の回転を表現するときは、「物体に固定された座標系」が「物体」の運動に従って回る、というプログラムにすることがある。この方が、たとえば図の飛行機の機首がにある、などと指定できて便利なこともあるのである。
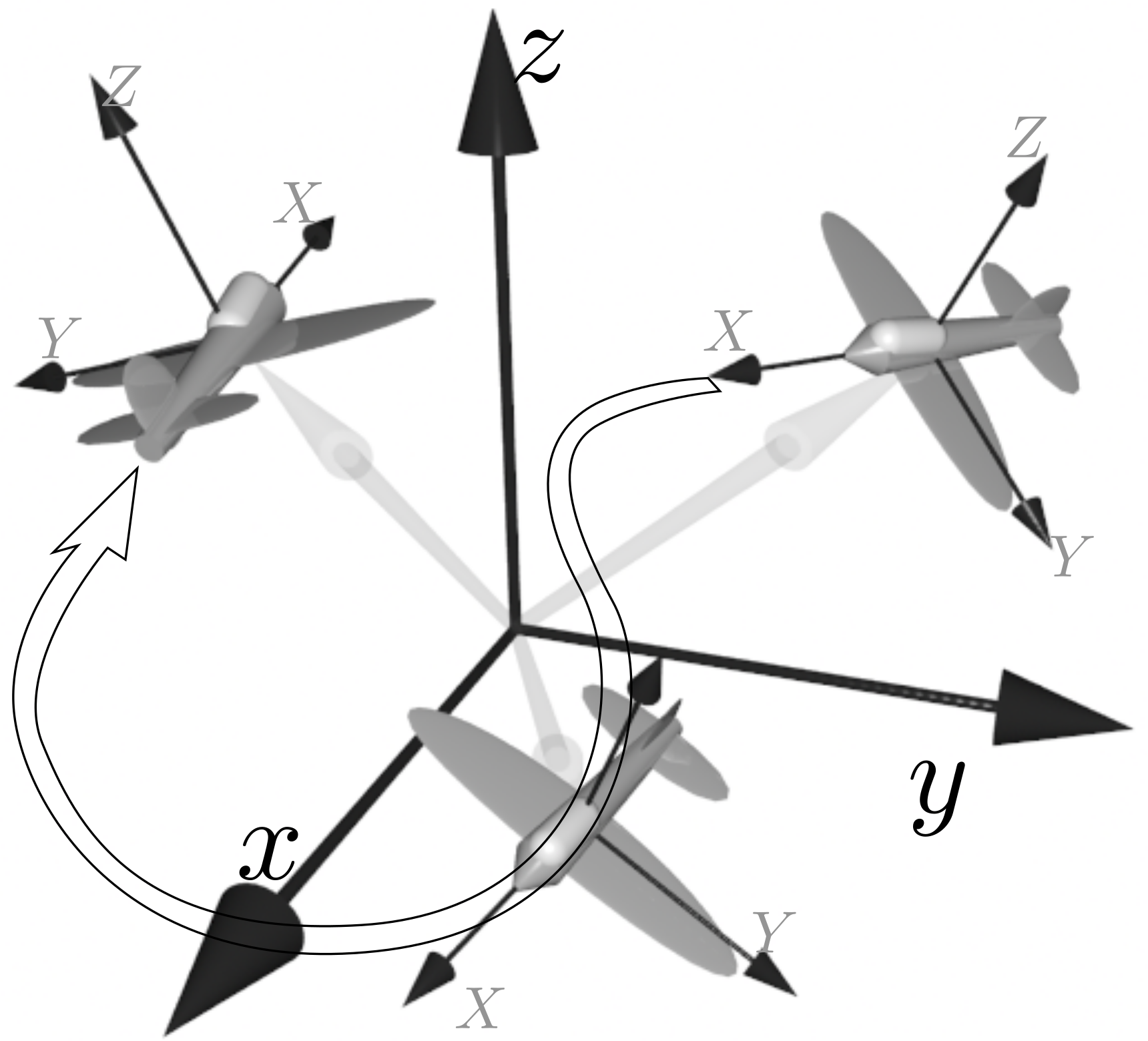
この場合は「(物体に固定された座標系の)基底の変換」の方が「(座標系がくくりつけられている)物体の回転」を表現して
いることになる。
テキストではこの後に「傾いた軸の周りの回転」の話があるが、授業ではそこは省略とする。各自読んでおいてください。
以上で第9回の授業は終わりです。webClassに行って、アンケートに答えてください。
物理数学I webclass
この感想・コメントシートに書かれたことについては、代表的なものに対しては次のページで返答します。
なお、webClassに情報を載せていますが、木と金の11:50〜12:50の間、オンラインオフィスアワーとしてzoomを開いてます。質問や相談などがある人は来て話してください。参加者が少ないので、物理系1年生向けのオフィスアワーと合同になってます。
受講者の感想・コメント
テイラー展開を行列で表せると知って驚きと感動を覚えた。行列が物理数学で応用がきくことの確認ができた。
演算子をテイラー展開したりするのは、この先の物理でも結構つかうと思います。
回転というのを物理で扱うとき、特に剛体が複雑に感じるなと思っていたが、行列を使うことでたくさんの式が一つにまとまってる感じがあるなと思った。複素数の話を行列と関連づけることで、複素数の世界をより理解した気になれた。
回転を表現するには、他にもいろんな方法がありますが、行列は一番シンプルな表現です。
転置によって成分の変換と基底の変換の二つのパターンができるのは面白いなと感じたし、どちらがどっちなのかは気を付けなければいけないなと思った。また、二次元極座標の微分と回転を表す行列が似ているように見えるが、これは極座標の微分もやっていることは同じということなのだろうか。
極座標の微分というのは、どれのことかな?θでの微分が回転の表現になっているのはその通りだと思います。
ベクトルを回転させるとき、行列をかけて基底ベクトルを変化させるという考え方があると知ることができました。基底の変換に使う行列と成分の変換に使う行列は、お互いに転置の関係であると実際に計算をしてみて納得しました。 sinやcosのテイラー展開を行列に代入したり、expに行列を代入するという発想は思いつかなかったです。行列もその他の変数と同じようにシグマでまとめる事ができると知って、面白いなと思いました。 ベクトルをx軸回りにθ回してからz軸回りにφ回すのと、z軸回りにφ回してからx軸回りにθ回すのでは、結果のベクトルが違っているという事をアプリを使って実感しました。その後、実際に行列を計算して、確かに行列の形が異なっているなと思いました。
expという関数は物理では非常によく出てくるんですが、それはこの関数が「微小な変化の積み重ね」を表現する形になっているからです。だから演算子や行列もexpの上に乗る。
回転行列を用いることにより3次元の様々な回転を表せられるのは便利だと思った。 「オイラー角」かっこいい名前だと思った。
オイラーさんはいろんなところに出てくるしね。
行列がテイラー展開でも使えたことにとても驚きました。今までの数学の知識がどんどんつながっていく感じがとても楽しかったです。
このあと、さらにつながっていくし、後の物理の科目のなかで線型代数に出会うことも多いと思います。
ベクトルの回転からテイラー展開して、expの定義と見比べることで微小回転の無限回繰り返しと表現できるのは、数学の柔軟さ、のようなものを実感できて面白いと感じた。生成子や3次元回転の表現などは今後も重要になってきそうで少し複雑な要素はしっかり復習して抑えておきたい。
微分・積分がうまく現象を表現できる例ですね。
今回は回転を行列で表すとどうなるかがわかり、成分の変化と基底ベクトルの変化で行列が変わることやそれらが転置の関係にある事、また転置の定理の4番目がなぜそうなるのかを実際に行列の掛け算を考えて、理解することが出来ました。
転置も後でよく使う、大事な手法です。
cosθ -sinθ sinθ cosθ で表せないのは 1 1 1 1 もですか?
かな? でもこれはそもそも直交行列じゃないから、「直交行列で、で表せないもの」の答えにはならないです。